

植物生态学报 ›› 2022, Vol. 46 ›› Issue (3): 300-310.DOI: 10.17521/cjpe.2021.0292
所属专题: 生态学研究的方法和技术; 生态系统碳水能量通量
黄樱, 陈挚, 石喆, 熊博文, 鄢春华*( ), 邱国玉*(
), 邱国玉*( )
)
收稿日期:2021-08-12
接受日期:2021-10-30
出版日期:2022-03-20
发布日期:2022-01-05
通讯作者:
鄢春华,邱国玉
作者简介:Qiu GY, qiugy@pkusz.edu.cn)基金资助:
HUANG Ying, CHEN Zhi, SHI Zhe, XIONG Bo-Wen, YAN Chun-Hua*( ), QIU Guo-Yu*(
), QIU Guo-Yu*( )
)
Received:2021-08-12
Accepted:2021-10-30
Online:2022-03-20
Published:2022-01-05
Contact:
YAN Chun-Hua,QIU Guo-Yu
Supported by:摘要:
蒸散发广义互补原理是实测数据稀少条件下估算蒸散发的重要方法, 其中准确估算参数αe是应用该方法的关键。该研究利用中国不同气候和生态类型的8个通量站数据, 首先基于实测数据校准得到αe年值及月值, 探究αe的时空变异性并对比使用不同时间尺度的αe对广义互补原理模型计算精度的影响。考虑到实际情况下蒸散发实测数据缺乏而无法校准得到αe, 进一步探究两个基于干旱系数(AI)的αe年值统计模型(下称Liu法和Brutsaert法)在站点尺度的适用性, 明确αe是否可以利用AI确定, 最后探讨各计算方法的误差来源。主要结论如下: 1)季节变化影响αe, 不同通量站αe月值变化规律有所差异; 在空间变化上, 湿润站点αe年值总体大于干旱站点。Liu法和Brutsaert法计算的αe接近年校准值。2)在应用广义互补原理模型时, 使用校准αe年值能取得较好的模拟精度, 使用各月份αe时精度进一步提升。两种基于AI的免校准方法取得较好的模拟效果, 当缺少实测数据而无法校准αe时, 基于AI计算αe具有较大的潜力。3)使用校准αe年值时广义互补原理模型能模拟出蒸散发的年内变化趋势, 但在部分月份估算值出现偏差。Liu法和Brutsaert法计算的蒸散发在干旱站点的夏季月份呈现低估现象, 原因可能在于高估了降雨集中的夏季月份的AI。结果也进一步验证了广义互补原理在估算广泛不同的自然环境下的蒸散发的潜力。
黄樱, 陈挚, 石喆, 熊博文, 鄢春华, 邱国玉. 蒸散发广义互补原理中关键参数αe的时空变化特征及计算方法分析. 植物生态学报, 2022, 46(3): 300-310. DOI: 10.17521/cjpe.2021.0292
HUANG Ying, CHEN Zhi, SHI Zhe, XIONG Bo-Wen, YAN Chun-Hua, QIU Guo-Yu. Temporal and spatial variation characteristics and different calculation methods for the key parameter αe in the generalized complementary principle of evapotranspiration. Chinese Journal of Plant Ecology, 2022, 46(3): 300-310. DOI: 10.17521/cjpe.2021.0292
| 生态系统类型 Ecosystem type | 通量站 Flux station | 下垫面类型 Underlying surface type | 气候类型 Climate type | 海拔 Altitude (m) | 经纬度 Longitude and latitude | 干旱系数 Aridity index | 研究年限 Research time |
|---|---|---|---|---|---|---|---|
| 森林 Forest | 鼎湖山 Dinghushan | 常绿阔叶林 Evergreen broadleaf forest | 亚热带季风湿润气候 Subtropical monsoon humid climate | 300 | 112.32° E 23.10° N | 0.85 | 2003-01-2010-12 |
| 西双版纳 Xishuangbanna | 热带雨林 Tropical rainforest | 热带季风气候 Tropical monsoon climate | 756 | 101.15° E 21.55° N | 0.73 | 2003-01-2010-12 | |
| 千烟洲 Qianyanzhou | 人工林 Artificial forest | 亚热带季风气候 Subtropical monsoon climate | 100 | 115.03° E 26.44° N | 1.02 | 2003-01- 2010-12 | |
| 长白山 Changbaishan | 针阔混交林 Mixed needleleaf and broadleaf forest | 温带大陆性气候 Temperate continental climate | 738 | 128.05° E 42.24° N | 1.92 | 2003-01-2010-12 | |
| 九寨沟 Jiuzhaigou | 针叶林 Coniferous forest | 高原温带季风气候 Plateau temperate monsoon climate | 2 478 | 103.87° E 33.15° N | 1.00 | 2013-08-2015-12 | |
| 草地 Grassland | 内蒙古 Nei Mongol | 温带典型草原 Typical temperate grassland | 温带半干旱草原气候 Temperate semi-arid steppe climate | 1 200 | 116.24° E 43.19° N | 3.96 | 2004-01-2010-12 |
| 海北 Haibei | 高寒草甸 Alpine meadow | 高原大陆性气候 Plateau continental climate | 3 190 | 101.19° E 37.39° N | 1.58 | 2003-01-2010-12 | |
| 农田 Farmland | 禹城 Yucheng | 旱作农田 Dry farmland | 温带季风半湿润气候 Temperate monsoon semi-humid climate | 28 | 116.34° E 36.49° N | 2.28 | 2003-01-2010-12 |
表1 中国通量站基本信息表
Table 1 Basic information table of the China flux sites
| 生态系统类型 Ecosystem type | 通量站 Flux station | 下垫面类型 Underlying surface type | 气候类型 Climate type | 海拔 Altitude (m) | 经纬度 Longitude and latitude | 干旱系数 Aridity index | 研究年限 Research time |
|---|---|---|---|---|---|---|---|
| 森林 Forest | 鼎湖山 Dinghushan | 常绿阔叶林 Evergreen broadleaf forest | 亚热带季风湿润气候 Subtropical monsoon humid climate | 300 | 112.32° E 23.10° N | 0.85 | 2003-01-2010-12 |
| 西双版纳 Xishuangbanna | 热带雨林 Tropical rainforest | 热带季风气候 Tropical monsoon climate | 756 | 101.15° E 21.55° N | 0.73 | 2003-01-2010-12 | |
| 千烟洲 Qianyanzhou | 人工林 Artificial forest | 亚热带季风气候 Subtropical monsoon climate | 100 | 115.03° E 26.44° N | 1.02 | 2003-01- 2010-12 | |
| 长白山 Changbaishan | 针阔混交林 Mixed needleleaf and broadleaf forest | 温带大陆性气候 Temperate continental climate | 738 | 128.05° E 42.24° N | 1.92 | 2003-01-2010-12 | |
| 九寨沟 Jiuzhaigou | 针叶林 Coniferous forest | 高原温带季风气候 Plateau temperate monsoon climate | 2 478 | 103.87° E 33.15° N | 1.00 | 2013-08-2015-12 | |
| 草地 Grassland | 内蒙古 Nei Mongol | 温带典型草原 Typical temperate grassland | 温带半干旱草原气候 Temperate semi-arid steppe climate | 1 200 | 116.24° E 43.19° N | 3.96 | 2004-01-2010-12 |
| 海北 Haibei | 高寒草甸 Alpine meadow | 高原大陆性气候 Plateau continental climate | 3 190 | 101.19° E 37.39° N | 1.58 | 2003-01-2010-12 | |
| 农田 Farmland | 禹城 Yucheng | 旱作农田 Dry farmland | 温带季风半湿润气候 Temperate monsoon semi-humid climate | 28 | 116.34° E 36.49° N | 2.28 | 2003-01-2010-12 |
| 方法 Method | 月份 Month | 鼎湖山 Dinghushan | 西双版纳 Xishuangbanna | 千烟洲 Qianyanzhou | 长白山 Changbaishan | 九寨沟 Jiuzhaigou | 海北 Haibei | 内蒙古 Nei Mongol | 禹城 Yucheng | |
|---|---|---|---|---|---|---|---|---|---|---|
| 校准法(年值) Calibration method (annual αe) | 1.03 | 1.16 | 1.07 | 1.11 | 1.11 | 1.04 | 0.92 | 1.42 | ||
| Liu法 Liu method | 1.18 | 1.20 | 1.15 | 1.05 | 1.15 | 1.08 | 0.95 | 1.02 | ||
| Brutsaert法 Brutsaert method | 1.07 | 1.10 | 1.03 | 0.89 | 1.04 | 0.94 | 0.71 | 0.85 | ||
| 校准法(月值) Calibration method (monthly αe) | 1 | 1.02 | 1.24 | 1.06 | - | 1.10 | 0.93 | - | - | |
| 2 | 0.96 | 1.29 | 1.07 | - | 1.06 | 0.86 | - | - | ||
| 3 | 0.96 | 1.16 | 1.06 | - | 1.00 | 0.85 | - | - | ||
| 4 | 1.04 | 1.06 | 1.08 | - | 1.00 | 0.92 | - | - | ||
| 5 | 1.04 | 1.06 | 1.09 | - | 1.15 | 0.92 | - | - | ||
| 6 | 1.01 | 1.17 | 1.12 | - | 1.15 | 1.04 | - | - | ||
| 7 | 0.98 | 1.19 | 1.09 | - | 1.11 | 1.16 | - | - | ||
| 8 | 1.00 | 1.19 | 1.03 | - | 1.10 | 1.17 | - | - | ||
| 9 | 1.04 | 1.13 | 1.02 | - | 1.20 | 1.08 | - | - | ||
| 10 | 1.11 | 1.14 | 1.05 | - | 1.19 | 0.90 | - | - | ||
| 11 | 1.18 | 1.16 | 1.07 | - | 1.20 | 0.89 | - | - | ||
| 12 | 1.18 | 1.18 | 1.08 | - | 1.20 | 0.99 | - | - | ||
表2 不同计算方法αe值对比
Table 2 Comparison of αe value of different methods
| 方法 Method | 月份 Month | 鼎湖山 Dinghushan | 西双版纳 Xishuangbanna | 千烟洲 Qianyanzhou | 长白山 Changbaishan | 九寨沟 Jiuzhaigou | 海北 Haibei | 内蒙古 Nei Mongol | 禹城 Yucheng | |
|---|---|---|---|---|---|---|---|---|---|---|
| 校准法(年值) Calibration method (annual αe) | 1.03 | 1.16 | 1.07 | 1.11 | 1.11 | 1.04 | 0.92 | 1.42 | ||
| Liu法 Liu method | 1.18 | 1.20 | 1.15 | 1.05 | 1.15 | 1.08 | 0.95 | 1.02 | ||
| Brutsaert法 Brutsaert method | 1.07 | 1.10 | 1.03 | 0.89 | 1.04 | 0.94 | 0.71 | 0.85 | ||
| 校准法(月值) Calibration method (monthly αe) | 1 | 1.02 | 1.24 | 1.06 | - | 1.10 | 0.93 | - | - | |
| 2 | 0.96 | 1.29 | 1.07 | - | 1.06 | 0.86 | - | - | ||
| 3 | 0.96 | 1.16 | 1.06 | - | 1.00 | 0.85 | - | - | ||
| 4 | 1.04 | 1.06 | 1.08 | - | 1.00 | 0.92 | - | - | ||
| 5 | 1.04 | 1.06 | 1.09 | - | 1.15 | 0.92 | - | - | ||
| 6 | 1.01 | 1.17 | 1.12 | - | 1.15 | 1.04 | - | - | ||
| 7 | 0.98 | 1.19 | 1.09 | - | 1.11 | 1.16 | - | - | ||
| 8 | 1.00 | 1.19 | 1.03 | - | 1.10 | 1.17 | - | - | ||
| 9 | 1.04 | 1.13 | 1.02 | - | 1.20 | 1.08 | - | - | ||
| 10 | 1.11 | 1.14 | 1.05 | - | 1.19 | 0.90 | - | - | ||
| 11 | 1.18 | 1.16 | 1.07 | - | 1.20 | 0.89 | - | - | ||
| 12 | 1.18 | 1.18 | 1.08 | - | 1.20 | 0.99 | - | - | ||
| 生态系统类型 Ecosystem type | 通量站 Flux station | 校准法(年值) Calibration method (annual value) | 校准法(月值) Calibration method (monthly value) | Brutsaert法 Brutsaert method (Brutsaert et al., | Liu法 Liu method (Liu et al., | ||||
|---|---|---|---|---|---|---|---|---|---|
| R2 | RMSE | R2 | RMSE | R2 | RMSE | R2 | RMSE | ||
| 森林 Forest | 鼎湖山 Dinghushan | 0.81 | 0.67 | 0.81 | 0.64 | 0.81 | 0.68 | 0.81 | 0.82 |
| 西双版纳 Xishuangbanna | 0.73 | 0.51 | 0.75 | 0.49 | 0.73 | 0.55 | 0.73 | 0.54 | |
| 千烟洲 Qianyanzhou | 0.92 | 0.55 | 0.92 | 0.54 | 0.91 | 0.60 | 0.91 | 0.64 | |
| 长白山 Changbaishan | 0.80 | 0.78 | - | - | 0.81 | 1.02 | 0.81 | 0.79 | |
| 九寨沟 Jiuzhaigou | 0.85 | 0.59 | 0.87 | 0.53 | 0.85 | 0.63 | 0.85 | 0.61 | |
| 草地 Grassland | 海北 Haibei | 0.92 | 0.45 | 0.96 | 0.34 | 0.92 | 0.54 | 0.92 | 0.46 |
| 内蒙古 Nei Mongol | 0.67 | 0.75 | - | - | 0.64 | 0.93 | 0.63 | 0.75 | |
| 农田 Farmland | 禹城 Yucheng | 0.78 | 0.97 | - | - | 0.76 | 1.82 | 0.77 | 1.45 |
表3 不同计算方法的均方根误差(RMSE)和决定系数(R2)
Table 3 Root mean square error (RMSE) and determinate coefficient (R2) of different calculation methods
| 生态系统类型 Ecosystem type | 通量站 Flux station | 校准法(年值) Calibration method (annual value) | 校准法(月值) Calibration method (monthly value) | Brutsaert法 Brutsaert method (Brutsaert et al., | Liu法 Liu method (Liu et al., | ||||
|---|---|---|---|---|---|---|---|---|---|
| R2 | RMSE | R2 | RMSE | R2 | RMSE | R2 | RMSE | ||
| 森林 Forest | 鼎湖山 Dinghushan | 0.81 | 0.67 | 0.81 | 0.64 | 0.81 | 0.68 | 0.81 | 0.82 |
| 西双版纳 Xishuangbanna | 0.73 | 0.51 | 0.75 | 0.49 | 0.73 | 0.55 | 0.73 | 0.54 | |
| 千烟洲 Qianyanzhou | 0.92 | 0.55 | 0.92 | 0.54 | 0.91 | 0.60 | 0.91 | 0.64 | |
| 长白山 Changbaishan | 0.80 | 0.78 | - | - | 0.81 | 1.02 | 0.81 | 0.79 | |
| 九寨沟 Jiuzhaigou | 0.85 | 0.59 | 0.87 | 0.53 | 0.85 | 0.63 | 0.85 | 0.61 | |
| 草地 Grassland | 海北 Haibei | 0.92 | 0.45 | 0.96 | 0.34 | 0.92 | 0.54 | 0.92 | 0.46 |
| 内蒙古 Nei Mongol | 0.67 | 0.75 | - | - | 0.64 | 0.93 | 0.63 | 0.75 | |
| 农田 Farmland | 禹城 Yucheng | 0.78 | 0.97 | - | - | 0.76 | 1.82 | 0.77 | 1.45 |
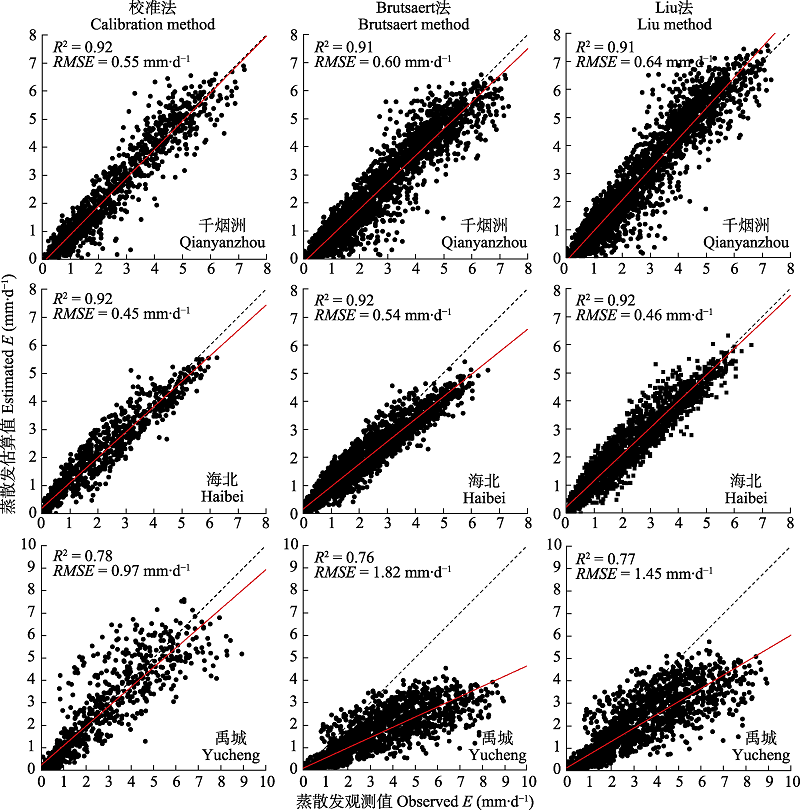
图1 互补原理日蒸散发估算值与观测值的比较结果。Liu法见文献Liu等(2016), Brutsaert法见文献Brutsaert等(2020)。RMSE, 均方根误差。
Fig. 1 Comparison of estimated daily evapotranspiration (E) calculated by the nonlinear complementary principle and observed E. Liu method refers to Liu et al. (2016), Brutsaert method refers to Brutsaert et al. (2020). RMSE, root mean square error.
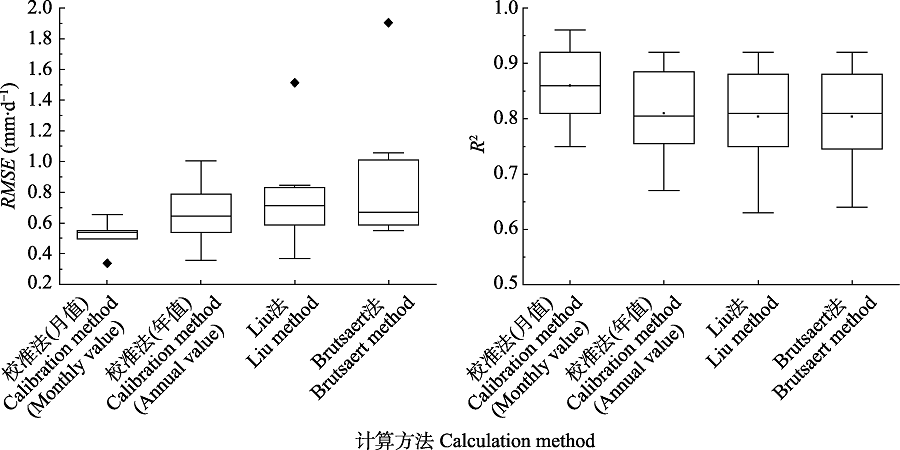
图2 不同计算方法的蒸散发估算值和观测值之间的均方根误差(RMSE)和决定系数(R2)箱线图。Liu法见文献Liu等(2016), Brutsaert法见文献Brutsaert等(2020)。
Fig. 2 Root mean square error (RMSE) and determinate coefficient (R2) boxplot between the estimated evapotranspiration (E) with different calculation methods and observed E at all stations. Liu method refers to Liu et al. (2016), Brutsaert method refers to Brutsaert et al. (2020).
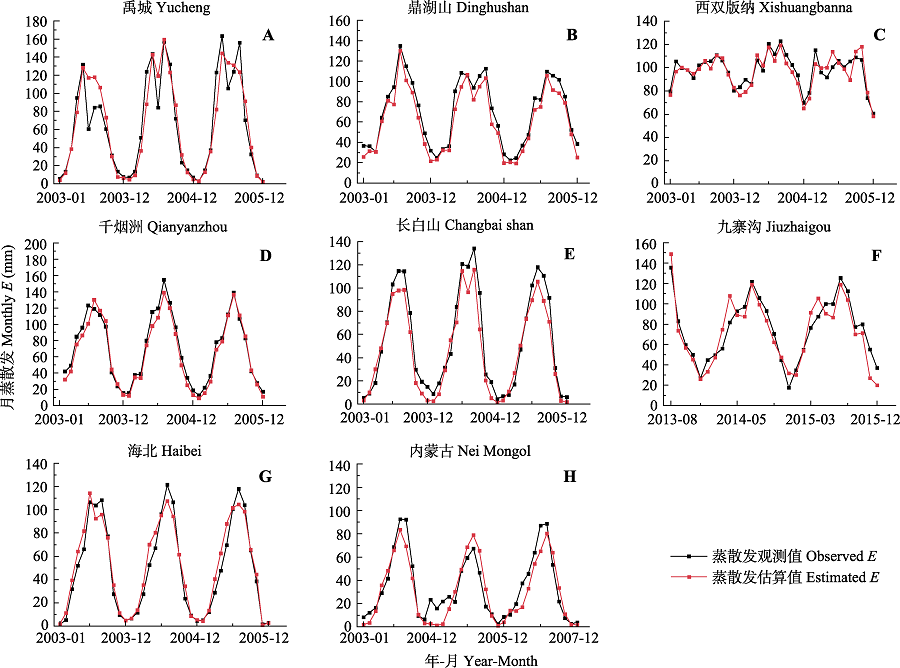
图3 由校准法确定αe年值, 广义互补原理估算的蒸散发月值与月蒸散发观测值结果对比。
Fig. 3 Comparison of monthly estimated evapotranspiration (E) calculated by calibration annual αe and observed E.
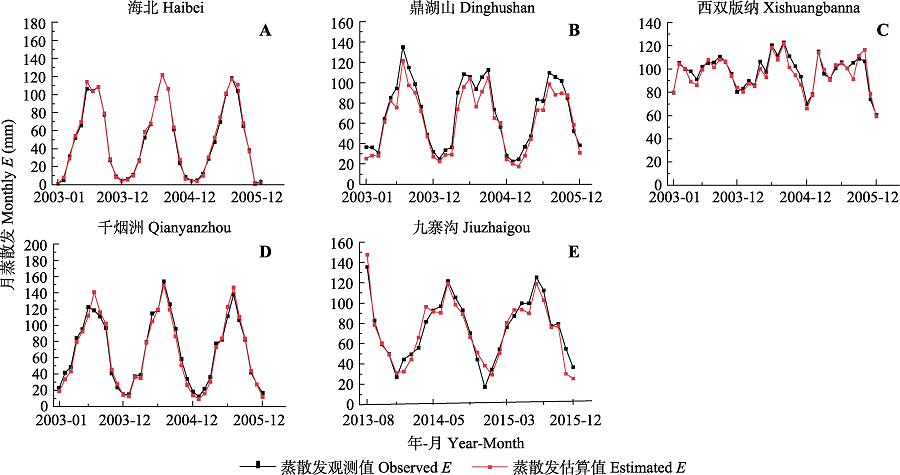
图4 由校准法确定各月αe, 广义互补原理估算的蒸散发月值与月蒸散发观测值结果对比。
Fig. 4 Comparison of monthly estimated evapotranspiration (E) calculated by calibration monthly αe and observed E.
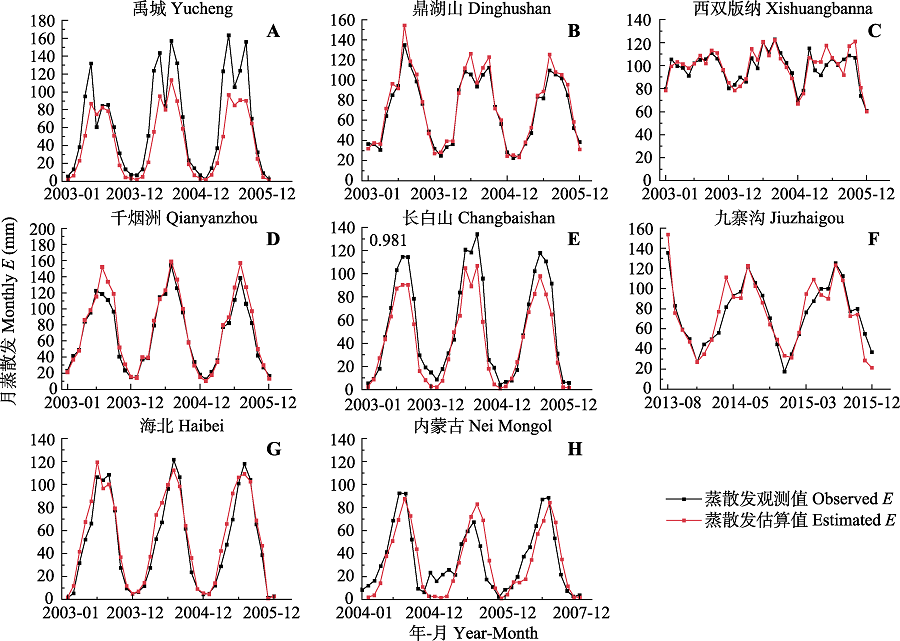
图5 由Liu法确定参数αe, 广义互补原理估算的蒸散发月值与月蒸散发观测值结果对比。Liu法见文献Liu等(2016)。
Fig. 5 Comparison of monthly estimated evapotranspiration (E) calculated by Liu method and observed E. Liu method refers to Liu et al. (2016).
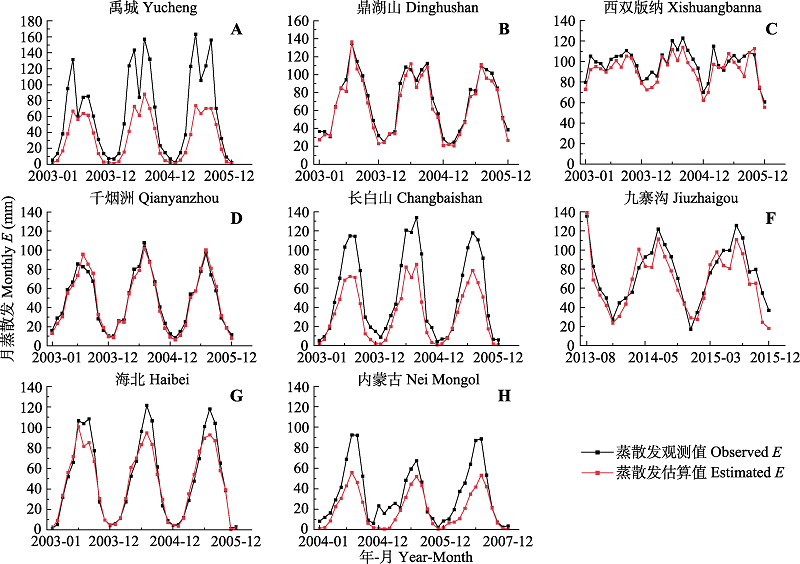
图6 由Brutsaert法确定参数αe, 广义互补原理估算的蒸散发月值与月蒸散发观测值结果对比。Brutsaert法见文献Brutsaert等(2020)。
Fig. 6 Comparison of monthly estimated evapotranspiration (E) calculated by Brutsaert method and observed E. Brutsaert method refers to Brutsaert et al. (2020)
| [1] |
Ai ZP, Wang QX, Yang YH, Manevski K, Zhao X, Eer DN (2017). Estimation of land-surface evaporation at four forest sites across Japan with the new nonlinear complementary method. Scientific Reports, 7, 17793. DOI: 10.1038/s41598-017-17473-0.
DOI URL |
| [2] | Allen RG, Pereira LS, Raes D, Smith M (1998). Crop Evapotranspiration: Guidelines for Computing Crop Water Requirements. FAO, Rome. |
| [3] |
Brutsaert W (2015). A generalized complementary principle with physical constraints for land-surface evaporation. Water Resources Research, 51, 8087-8093.
DOI URL |
| [4] |
Brutsaert W, Li W, Takahashi A, Hiyama T, Zhang L, Liu WZ (2017). Nonlinear advection-aridity method for landscape evaporation and its application during the growing season in the southern Loess Plateau of the Yellow River basin. Water Resources Research, 53, 270-282.
DOI URL |
| [5] |
Brutsaert W, Cheng L, Zhang L (2020). Spatial distribution of global landscape evaporation in the early twenty-first century by means of a generalized complementary approach. Journal of Hydrometeorology, 21, 287-298.
DOI URL |
| [6] |
Han SJ, Hu HP, Tian FQ (2012). A nonlinear function approach for the normalized complementary relationship evaporation model. Hydrological Processes, 26, 3973-3981.
DOI URL |
| [7] |
Han SJ, Tian FQ (2018). Derivation of a sigmoid generalized complementary function for evaporation with physical constraints. Water Resources Research, 54, 1734-1736.
DOI URL |
| [8] |
Hu ZY, Wang GX, Sun XY, Zhu MZ, Song CL, Huang KW, Chen XP (2018). Spatial-temporal patterns of evapotranspiration along an elevation gradient on mount Gongga, southwest China. Water Resources Research, 54, 4180-4192.
DOI URL |
| [9] | Jian DN, Li XC, Tao H, Huang JL, Su BD (2016). Spatio- temporal variation of actual evapotranspiration and its influence factors in the Tarim River basin based on the complementary relationship approach. Journal of Glaciology and Geocryology, 38, 750-760. |
| [蹇东南, 李修仓, 陶辉, 黄金龙, 苏布达 (2016). 基于互补相关理论的塔里木河流域实际蒸散发时空变化及影响因素分析. 冰川冻土, 38, 750-760.] | |
| [10] |
Li TS, Xia J, Zhang L, She DX, Wang GS, Cheng L (2021). An improved complementary relationship for estimating evapotranspiration attributed to climate change and revegetation in the Loess Plateau, China. Journal of Hydrology, 592, 125516. DOI: 10.1016/j.jhydrol.2020.125516.
DOI URL |
| [11] | Li Q, Cheng L, Ye LY, Liu P, Xiong LH (2020). Long-term land surface evaporation and its changes estimated by the generalized complementary principle in China. Journal of Water Resources Research, 9, 259-269. |
| [李曲, 程磊, 叶林媛, 刘攀, 熊立华 (2020). 基于广义蒸发互补关系的中国长期陆面蒸发量及其变化分析. 水资源研究, 9, 259-269.] | |
| [12] | Liu X, Liu C, Brutsaert W (2018). Investigation of a generalized nonlinear form of the complementary principle for evaporation estimation. Journal of Geophysical Research, 123, 3933-3942. |
| [13] |
Liu XM, Liu CM, Brutsaert W (2016). Regional evaporation estimates in the eastern monsoon region of China: assessment of a nonlinear formulation of the complementary principle. Water Resources Research, 52, 9511-9521.
DOI URL |
| [14] | Penman HL (1948). Natural Evaporation from open water, bare soil and grass. Proceedings of the Royal Society of London, Series A, Mathematical and Physical Sciences, 193, 120-145. |
| [15] | Slatyer RO, Mcilroy IC (1961). Practical Microclimatology. CSIRO, Melbourne. |
| [16] | Szilagyi J, Crago R, Qualls R (2017). A calibration-free formulation of the complementary relationship of evaporation for continental-scale hydrology. Journal of Geophysical Research, 122, 264-278. |
| [17] |
Wang LM, Han SJ, Tian FQ (2021). At which timescale does the complementary principle perform best in evaporation estimation? Hydrology and Earth System Sciences, 25, 375-386.
DOI URL |
| [18] | Wen SS, Jiang T, Li XC, Wang TF, Wang YJ, Fischer T (2014). Changes of actual evapotranspiration over the Songhua River basin from 1961 to 2010. Climate Change Research, 10(2), 79-86. |
| [温姗姗, 姜彤, 李修仓, 王腾飞, 王艳君, Fischer T (2014). 1961-2010年松花江流域实际蒸散发时空变化及影响要素分析. 气候变化研究进展, 10(2), 79-86.] | |
| [19] |
Yan CH, Zhao WL, Wang Y, Yang QX, Zhang QT, Qiu GY (2017). Effects of forest evapotranspiration on soil water budget and energy flux partitioning in a subalpine valley of China. Agricultural and Forest Meteorology, 246, 207-217.
DOI URL |
| [20] |
Yang DW, Shao WW, Yeh PJF, Yang HB, Kanae S, Oki T (2009). Impact of vegetation coverage on regional water balance in the nonhumid regions of China. Water Resources Research, 45, W00A14. DOI: 10.1029/2008WR006948.
DOI |
| [21] |
Yang HB, Yang DW, Lei ZD (2013). Seasonal variability of the complementary relationship in the Asian monsoon region. Hydrological Processes, 27, 2736-2741.
DOI URL |
| [22] | Zhang L, Cheng L, Brutsaert W (2017). Estimation of land surface evaporation using a generalized nonlinear complementary relationship. Journal of Geophysical Research, 122, 1475-1487. |
| [23] | Zhao YM (2019). Analysis of Estimating and Driving Force of Actual Evapotranspiration in Watershed Based on Generalized Complementary Relationship. Nanjing University of Information Science & Technology, Nanjing. |
| [赵宇铭 (2019). 基于广义互补相关理论的流域实际蒸散发估算及驱动力分析. 南京信息工程大学, 南京.] |
| [1] | 冯印成, 王云琦, 王玉杰, 王凯, 王松年, 王杰帅. 重庆缙云山针阔混交林水汽通量特征及其影响因子[J]. 植物生态学报, 2022, 46(8): 890-903. |
| [2] | 王彦兵, 游翠海, 谭星儒, 陈波宇, 许梦真, 陈世苹. 中国北方干旱半干旱区草原生态系统能量平衡闭合的季节和年际变异[J]. 植物生态学报, 2022, 46(12): 1448-1460. |
| [3] | 林雍, 陈智, 杨萌, 陈世苹, 高艳红, 刘冉, 郝彦宾, 辛晓平, 周莉, 于贵瑞. 中国干旱半干旱区生态系统光合参数的时空变异及其影响因素[J]. 植物生态学报, 2022, 46(12): 1461-1472. |
| [4] | 李红琴, 张亚茹, 张法伟, 马文婧, 罗方林, 王春雨, 杨永胜, 张雷明, 李英年. 增强回归树模型在青藏高原高寒灌丛通量数据插补中的应用[J]. 植物生态学报, 2022, 46(12): 1437-1447. |
| [5] | 陈世苹, 游翠海, 胡中民, 陈智, 张雷明, 王秋凤. 涡度相关技术及其在陆地生态系统通量研究中的应用[J]. 植物生态学报, 2020, 44(4): 291-304. |
| [6] | 柴曦, 李英年, 段呈, 张涛, 宗宁, 石培礼, 何永涛, 张宪洲. 青藏高原高寒灌丛草甸和草原化草甸CO2通量动态及其限制因子[J]. 植物生态学报, 2018, 42(1): 6-19. |
| [7] | 徐静馨, 郑有飞, 麦博儒, 赵辉, 储仲芳, 黄积庆, 袁月. 基于涡度相关法的麦田O3干沉降及不同沉降通道分配的特征[J]. 植物生态学报, 2017, 41(6): 670-682. |
| [8] | 王玉辉, 井长青, 白洁, 李龙辉, 陈曦, 罗格平. 亚洲中部干旱区3个典型生态系统生长季水碳通量特征[J]. 植物生态学报, 2014, 38(8): 795-808. |
| [9] | 张峰, 周广胜. 玉米农田冠层光合参数的多光谱遥感反演[J]. 植物生态学报, 2014, 38(7): 710-719. |
| [10] | 杨利琼,韩广轩,于君宝,吴立新,朱敏,邢庆会,王光美,毛培利. 开垦对黄河三角洲湿地净生态系统CO2交换的影响[J]. 植物生态学报, 2013, 37(6): 503-516. |
| [11] | 张法伟, 李英年, 曹广民, 李凤霞, 叶广继, 刘吉宏, 魏永林, 赵新全. 青海湖北岸高寒草甸草原生态系统CO2通量特征及其驱动因子[J]. 植物生态学报, 2012, 36(3): 187-198. |
| [12] | 吴力博, 古松, 赵亮, 徐世晓, 周华坤, 冯超, 徐维新, 李英年, 赵新全, 唐艳鸿. 三江源地区人工草地的生态系统CO2净交换、总初级生产力及其影响因子[J]. 植物生态学报, 2010, 34(7): 770-780. |
| [13] | 刘晨峰, 张志强, 孙阁, 查同刚, 朱金兆, 申李华, 陈军, 方显瑞, 陈吉泉. 基于涡度相关法和树干液流法评价杨树人工林 生态系统蒸发散及其环境响应[J]. 植物生态学报, 2009, 33(4): 706-718. |
| [14] | 顾峰雪, 于贵瑞, 温学发, 陶波, 李克让, 刘允芬. 干旱对亚热带人工针叶林碳交换的影响[J]. 植物生态学报, 2008, 32(5): 1041-1051. |
| [15] | 黄祥忠, 郝彦宾, 王艳芬, 周小奇, 韩喜, 贺俊杰. 极端干旱条件下锡林河流域羊草草原净生态系统碳交换特征[J]. 植物生态学报, 2006, 30(6): 894-900. |
| 阅读次数 | ||||||
|
全文 |
|
|||||
|
摘要 |
|
|||||
Copyright © 2022 版权所有 《植物生态学报》编辑部
地址: 北京香山南辛村20号, 邮编: 100093
Tel.: 010-62836134, 62836138; Fax: 010-82599431; E-mail: apes@ibcas.ac.cn, cjpe@ibcas.ac.cn
备案号: 京ICP备16067583号-19